Nano-mechanical-oscillator coupled by Bose Einstein
Condensates (NanoBEC)
Hybrid quantum mechanical system
Laser light has been successfully used to trap cold atoms or even cool the atoms to the quantum ground state (BEC) due to its nature of radiation pressure and dipole forces. Nowadays, it is also believed that it could also be used to cool a macroscopic object, such as a mechanical oscillator in a special mode to the quantum ground state using the same cooling method. The radiation pressure force has already been solely used to cool different mechanical oscillators to approach the quantum ground states using either passive or active cooling schemes. The main bottlenecks for pure optical ways are the low cooling efficiency (especially for kHz oscillators) as well as the detecting difficulties for the zero-point-fluctuations especially when the mechanical oscillator is approaching the mode ground state (Tmode~0 K). The cooling efficiency can be highly increased if one can precool the mechanical oscillator to cryogenic temperature (30 mK for example) from room temperature (273 K). In this case the mean phonon occupation number of the cantilever (i.e., assuming a 10 kHz natural frequency) can be reduced from 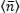 = 108 to 1014. The detecting sensitivity can also be improved by designing a high finesse low noise interferometer. For a kHz mechanical oscillator, in order to detect the zero-point-fluctuation, the resolution of the detecting/readout system has to be improved to be in sub-pm / √Hz or even fm / √Hz regime.
= 108 to 1014. The detecting sensitivity can also be improved by designing a high finesse low noise interferometer. For a kHz mechanical oscillator, in order to detect the zero-point-fluctuation, the resolution of the detecting/readout system has to be improved to be in sub-pm / √Hz or even fm / √Hz regime.
A better option to cool the mechanical oscillator is to use a hybrid quantum mechanical system, the working principle can be simply explained as follows (also see figure 1): a mechanical oscillator, i.e., an AFM cantilever (CL) or a membrane with a natural resonance frequency of 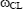 , can used as a flexible ending mirror to form a 1D standing wave. Cold atoms (i.e., 87Rb) trapping inside the standing wave, called optical lattice, have a axial trapping frequency
, can used as a flexible ending mirror to form a 1D standing wave. Cold atoms (i.e., 87Rb) trapping inside the standing wave, called optical lattice, have a axial trapping frequency 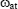 . The flexural movement of the cantilever i.e. due to the thermal fluctuation will modulate the standing wave, which will causes collective harmonic oscillation of the center of mass of the cold atoms trapped inside the optical lattice. The optical trap will generate a dipole restoring force to the cold atoms, and its reaction will inversely redistribute the photons in the standing wave by generating a phase lag and thus dissipate the oscillating energy of the cantilever. When resonant coupling happens, that is
. The flexural movement of the cantilever i.e. due to the thermal fluctuation will modulate the standing wave, which will causes collective harmonic oscillation of the center of mass of the cold atoms trapped inside the optical lattice. The optical trap will generate a dipole restoring force to the cold atoms, and its reaction will inversely redistribute the photons in the standing wave by generating a phase lag and thus dissipate the oscillating energy of the cantilever. When resonant coupling happens, that is 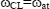 , energy transfer will happen between the two different mechanical systems. Hence, removing energy from the trapped atom will decrease the total energy in the coupled system and sympathetically cool the mode of the cantilever to quantum ground state with a mean phonon occupation number of
, energy transfer will happen between the two different mechanical systems. Hence, removing energy from the trapped atom will decrease the total energy in the coupled system and sympathetically cool the mode of the cantilever to quantum ground state with a mean phonon occupation number of 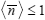 .
.
Figure 1. (a) The kHz mechanical oscillator i.e. an AFM cantilever (CL) can be firstly precooled by the cold finger of the dilution refrigerator at around 30 mK in order to bring down the phonon number to a few thousands. The successive cooling of cantilever mode can then be achieved by coupling the cantilever with a natural flexural frequency of 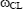 , to a 1 D optical lattice inside a glass cell where cold atoms are trapped inside with a harmonic trapping frequency of
, to a 1 D optical lattice inside a glass cell where cold atoms are trapped inside with a harmonic trapping frequency of 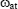 due to the optical dipole force (insert: pancake microtraps for 1D optical lattice, tight trapping along symmetrical axis). The two mechanical systems are mediated by the standing wave from a cooling laser beam (purple) formed between one movable ending 'mirror' of the CL and the other fixed retroreflective ending mirror. When
due to the optical dipole force (insert: pancake microtraps for 1D optical lattice, tight trapping along symmetrical axis). The two mechanical systems are mediated by the standing wave from a cooling laser beam (purple) formed between one movable ending 'mirror' of the CL and the other fixed retroreflective ending mirror. When 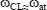 , the sympathetical cooling of the cantilever can be achieved by removing energy from the trapped atoms in the lattice in this strongly coupled hybrid quantum system. The damped motion of the CL can be detected using a second laser beam (light blue) through a high sensitive low noise detector (b) The standing wave (green) of light field is modulated back (brown) and forth (purple) due to the movement of the flexible cantilever, generating a harmonic potential sensed by the trapped atoms. The back action of the dipole force to the light field could inversely redistribute the photons in the reflected lattice beams and produce a phase lag to the radiation pressure used to damp out the motion of the cantilever.
, the sympathetical cooling of the cantilever can be achieved by removing energy from the trapped atoms in the lattice in this strongly coupled hybrid quantum system. The damped motion of the CL can be detected using a second laser beam (light blue) through a high sensitive low noise detector (b) The standing wave (green) of light field is modulated back (brown) and forth (purple) due to the movement of the flexible cantilever, generating a harmonic potential sensed by the trapped atoms. The back action of the dipole force to the light field could inversely redistribute the photons in the reflected lattice beams and produce a phase lag to the radiation pressure used to damp out the motion of the cantilever.

